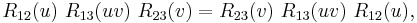Yang–Baxter equation
The Yang–Baxter equation (or star-triangle relation) is an equation which was first introduced in the field of statistical mechanics. It takes its name from independent work of C. N. Yang from 1968, and R. J. Baxter from 1971. It refers to a principle in integrable systems taking the form of local equivalence transformations which appear in a variety of contexts, such as electric networks, knot theory and braid groups, and spin systems, to name just a few.
Contents |
Parameter-dependent Yang–Baxter equation
Let  be a unital associative algebra. The parameter-dependent Yang–Baxter equation is an equation for
be a unital associative algebra. The parameter-dependent Yang–Baxter equation is an equation for 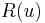 , a parameter-dependent invertible element of the tensor product
, a parameter-dependent invertible element of the tensor product  (here,
(here,  is the parameter, which usually ranges over all real numbers in the case of an additive parameter, or over all positive real numbers in the case of a multiplicative parameter). The Yang–Baxter equation is
is the parameter, which usually ranges over all real numbers in the case of an additive parameter, or over all positive real numbers in the case of a multiplicative parameter). The Yang–Baxter equation is
for all values of  and
and 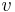 , in the case of an additive parameter. At some value of the parameter
, in the case of an additive parameter. At some value of the parameter 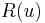 can turn into one dimensional projector, this gives rise to quantum determinant. For multiplicative parameter Yang–Baxter equation is
can turn into one dimensional projector, this gives rise to quantum determinant. For multiplicative parameter Yang–Baxter equation is
for all values of  and
and 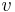 , where
, where 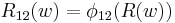 ,
, 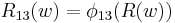 , and
, and  , for all values of the parameter
, for all values of the parameter 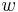 , and
, and 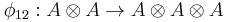 ,
, 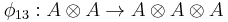 , and
, and 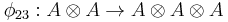 , are algebra morphisms determined by
, are algebra morphisms determined by
In some cases the determinant of 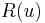 can vanish at specific values of the spectral parameter
can vanish at specific values of the spectral parameter  . Some
. Some  matrices turn into one dimensional projector at
matrices turn into one dimensional projector at  . In this case quantum determinant can be defined.
. In this case quantum determinant can be defined.
Parameter-independent Yang–Baxter equation
Let  be a unital associative algebra. The parameter-independent Yang–Baxter equation is an equation for
be a unital associative algebra. The parameter-independent Yang–Baxter equation is an equation for  , an invertible element of the tensor product
, an invertible element of the tensor product  . The Yang–Baxter equation is
. The Yang–Baxter equation is
where 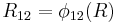 ,
, 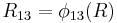 , and
, and  .
.
Let  be a module of
be a module of  . Let
. Let 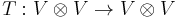 be the linear map satisfying
be the linear map satisfying  for all
for all 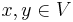 . Then a representation of the braid group,
. Then a representation of the braid group, 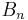 , can be constructed on
, can be constructed on 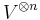 by
by  for
for  , where
, where 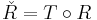 on
on  . This representation can be used to determine quasi-invariants of braids, knots and links.
. This representation can be used to determine quasi-invariants of braids, knots and links.
See also
References
- H.-D. Doebner, J.-D. Hennig, eds, Quantum groups, Proceedings of the 8th International Workshop on Mathematical Physics, Arnold Sommerfeld Institute, Clausthal, FRG, 1989, Springer-Verlag Berlin, ISBN 3-540-53503-9.
- Vyjayanthi Chari and Andrew Pressley, A Guide to Quantum Groups, (1994), Cambridge University Press, Cambridge ISBN 0-521-55884-0.
- Jacques H.H. Perk and Helen Au-Yang, "Yang–Baxter Equations", (2006), arXiv:math-ph/0606053.